1102. Sticks problem
Xuanxuan has n sticks of different length. One
day, she puts all her sticks in a line, represented by s1, s2, s3, ..., sn. After measuring the length of each stick sk (1 ≤ k ≤ n),
she finds that for some sticks si and sj (1 ≤ i < j ≤ n), each stick placed
between si and sj is longer than si but shorter than sj.
Now given the length of s1, s2, s3, ..., sn, you are required to find the maximum value j – i.
Input. Contains
multiple test cases. Each case contains two lines. First line is a single
integer n (n ≤ 50000), indicating the number of sticks. Second line
contains n different positive integers (not larger than 105), indicating the length of each stick
in order.
Output. Output
the maximum value j – i in a single
line. If there is no such i and j, just output -1.
|
Sample input |
Sample output |
|
4 5 4 3 6 4 6 5 4 3 9 12 4 8 7 5 9
6 3 1 |
1 -1 4 |
SOLUTION
RMQ
+ binary search
Algorithm analysis
For each index of i, find the
maximum index k (i ≤ k ≤ n) for which
RMinQ(si+1,
…, sk) > si with binary search. The desired j is the index at
which RMaxQ(si,
…, sk) (i ≤ j ≤ k) is achieved.
Thus, for each index of i, find the
maximum possible index j and among all such pairs (i, j) calculate the maximum difference j – i.
Example
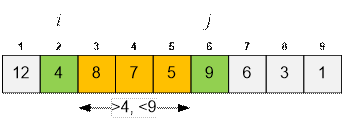
Consider the third sample.
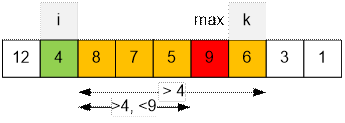
Let i = 2 (s2 = 4). The largest index is k = 7, for which RMinQ(si+1,
…, sk) > 4. RMaxQ(s3, …, s7) is achieved at index j = 6.
Algorithm
realization
#include <cstdio>
#include <algorithm>
#define MAX 50010
#define LOGMAX 16
using namespace std;
int dp_max[MAX][LOGMAX], dp_min[MAX][LOGMAX];
int a[MAX];
int i, j, n, res;
void Build_RMQ_Array(int
*b)
{
int i, j;
for (i = 1; i
<= n; i++)
{
dp_min[i][0] = b[i];
dp_max[i][0] = i;
}
for (j = 1; 1
<< j <= n; j++)
for (i = 1;
i + (1 << j) - 1 <= n; i++)
{
if
(b[dp_max[i][j - 1]] > b[dp_max[i + (1 << (j - 1))][j - 1]])
dp_max[i][j] = dp_max[i][j - 1];
else
dp_max[i][j] = dp_max[i + (1 <<
(j - 1))][j - 1];
dp_min[i][j] =
min(dp_min[i][j - 1], dp_min[i + (1
<< (j - 1))][j - 1]);
}
}
int RangeMaxQuery(int
i, int j)
{
int k = 0;
while ((1
<< (k + 1)) <= j - i + 1) k++;
if
(a[dp_max[i][k]] > a[dp_max[j - (1<<k) + 1][k]])
return dp_max[i][k];
else
return dp_max[j - (1<<k) + 1][k];
}
int RangeMinQuery(int
i, int j)
{
int k = 0;
while ((1
<< (k + 1)) <= j - i + 1) k++;
return
min(dp_min[i][k],dp_min[j - (1<<k) + 1][k]);
}
int BinSearch(int
Left, int Right)
{
int MinValue
= a[Left++];
if
(RangeMinQuery(Left,Right) > MinValue) return
Right;
while (Right
> Left)
{
int Middle
= (Left + Right) / 2;
if
(RangeMinQuery(Left,Middle) > MinValue)
Left = Middle + 1;
else
Right = Middle;
}
if (dp_min[Left][0]
<= MinValue) Left--;
return Left;
}
int main(void)
{
while(scanf("%d",&n) == 1)
{
for(i = 1;
i <= n; i++) scanf("%d",&a[i]);
Build_RMQ_Array(a);
res = 0;
for(i = 1;
i <= n; i++)
{
j = BinSearch(i, n);
j =
RangeMaxQuery(i,j);
if (j - i
> res) res = j - i;
}
if (res ==
0) res = -1;
printf("%d\n",res);
}
return 0;
}